Earthquakes are natural disasters that have fascinated, puzzled, and terrified humanity for centuries. To comprehend the severity and potential destruction they can cause, scientists developed various methods of measuring earthquake magnitude. One of the most renowned and widely used scales for this purpose is the Richter Scale. In this blog, we will explore the Richter Scale, its history, how it works, and its significance in assessing earthquake intensity.
The Birth of the Richter Scale
The Richter Scale, also known as the local magnitude scale (ML), was developed by Charles F. Richter and Beno Gutenberg in 1935. Charles Richter was a seismologist and mathematician, while Beno Gutenberg was a renowned seismologist. Together, they sought to create a standardized way of quantifying the size or magnitude of earthquakes. Their collaboration led to the birth of the Richter Scale, a logarithmic scale that revolutionized the field of seismology.
How Does the Richter Scale Work?
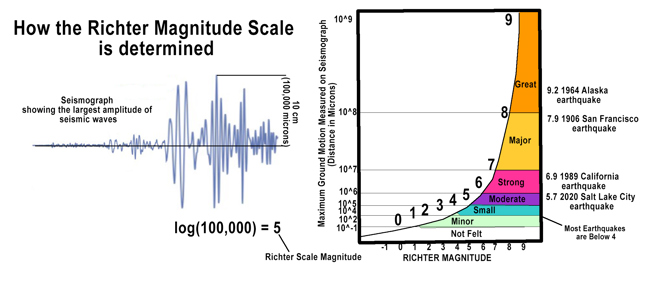
The Richter Scale is designed to measure the energy released by an earthquake at its source. It uses a logarithmic scale, meaning that each whole number increase on the Richter Scale represents a tenfold increase in the amplitude of seismic waves and approximately 31.6 times more energy release. This logarithmic nature allows the Richter Scale to effectively capture the vast range of earthquake magnitudes, from minor tremors to major quakes.
Here's how it works:
-
Seismic Sensors:
Seismometers, or seismographs, are placed strategically around the world to detect and record ground motion caused by earthquakes. -
Recording Data:
When an earthquake occurs, the seismometers detect the ground motion and generate a seismogram, which is a graphical representation of the seismic waves produced by the earthquake. -
Amplitude Measurement:
The amplitude of the seismic waves on the seismogram is measured. This amplitude represents the maximum ground displacement caused by the earthquake. -
Logarithmic Calculation:
Using the measured amplitude, the Richter Scale calculates the earthquake's magnitude using the following formula:ML = log10(A/T) + constant
Where:
- ML is the Richter magnitude
- A is the maximum amplitude recorded on the seismogram
- T is the period of the seismic waves
- The constant is used for calibration purposes
-
Reporting the Magnitude:
The resulting Richter magnitude is then used to report the earthquake's size or intensity.
Logarithmic Nature of the Richter Scale, Each Magnitude is Tenfold Greater 😲
The Richter Scale is a logarithmic scale, which means it quantifies measurements using a logarithm to the base 10. In this context, the base 10 logarithm allows scientists to express a wide range of values in a more manageable and comprehensible way. This approach is particularly crucial when dealing with earthquakes because they can vary greatly in intensity.
So, Magnitude (M2) = 10 x Magnitude (M1) and so on!
Curated Reference Video:
Significance of the Richter Scale
The Richter Scale has been instrumental in several aspects of earthquake science and preparedness:
-
Comparing Earthquakes: The scale provides a standardized way to compare the magnitudes of different earthquakes. A magnitude 5 earthquake is ten times larger in amplitude and 31.6 times more energetic than a magnitude 4 earthquake.
-
Early Warning Systems: By quickly determining the magnitude of an earthquake, authorities can issue timely warnings to affected areas, potentially saving lives and minimizing damage.
-
Historical Analysis: The Richter Scale allows scientists to analyze and compare earthquake data from the past, aiding in understanding seismic patterns and risk assessment in specific regions.
-
Engineering and Construction: Engineers and architects use seismic data, including Richter magnitudes, to design and construct buildings and infrastructure that can withstand earthquake forces.
Limitations of the Richter Scale
While the Richter Scale has been invaluable in the study of earthquakes, it does have some limitations:
-
Saturation:
The Richter Scale can become saturated for very large earthquakes, providing less precise estimates of their magnitudes. For such events, seismologists often use the moment magnitude scale (Mw) for greater accuracy. -
Distance Dependency:
The Richter Scale measures the magnitude of an earthquake at its source. It doesn't take into account the distance from the epicenter, which can influence the intensity experienced at different locations.
Classification by Magnitude:
| Magnitude | Effects | Description |
|---|---|---|
| 1.0 - 2.9 | Microearthquake | Usually not felt by people; detected only by seismographs. |
| 3.0 - 3.9 | Minor earthquake | Often felt but rarely causes damage. |
| 4.0 - 4.9 | Light earthquake | Noticeable shaking of indoor items, but rarely causes damage. |
| 5.0 - 5.9 | Moderate earthquake | Can cause damage to buildings and other structures. |
| 6.0 - 6.9 | Strong earthquake | Can cause significant damage in populated areas. |
| 7.0 - 7.9 | Major earthquake | Widespread and severe structural damage likely. |
| 8.0 - 8.9 | Great earthquake | Can cause massive damage in areas several tens of miles across. |
| 9.0 - 9.9 | Rare great earthquake | Devastating in areas several hundred miles across. |
| 10.0+ | Legendary "Mythical" Earthquake | Hypothetical; would have global impacts and destruction. |
Conclusion
The Richter Scale, developed by Charles Richter and Beno Gutenberg, revolutionized our ability to measure and understand earthquakes. Its logarithmic nature allows us to capture a wide range of magnitudes, from minor tremors to major quakes, and its significance extends to earthquake preparedness, engineering, and historical analysis. While it has some limitations, it remains a valuable tool in the field of seismology, helping us better comprehend and mitigate the destructive power of earthquakes.
Discover More
Most Viewed
Nature never ceases to amaze us with its breathtaking beauty and natural phenomena. In this blog, we highlight ten fascinating natural events that attract travelers from around the world.
Read More
